To find the area of the minor segment and major sector of a circle with a radius of 10 cm, where the chord subtends a right angle at the center:
Minor Segment: The area of the minor segment is the area of the sector minus the area of the triangle formed by the radii and the chord. The sector’s angle is 90°, so its area is (90/360)×π×10² = 25π cm². The triangle is a right-angled isosceles triangle (45-45-90), so its area is (1/2)×10×10 = 50 cm². The minor segment’s area is 25π − 50 cm².
Major Sector: The major sector is the rest of the circle, so its area is the total area of the circle minus the minor sector’s area. The circle’s area is π×10² = 100π cm². Therefore, the major sector’s area is 100π − 25π = 75π cm².
Let’s discuss in detail
Introduction to Circle Segments and Sectors
The problem of finding the areas of specific segments and sectors in a circle is a classic example of applying geometric principles to solve real-world problems. In this case, we are dealing with a circle of radius 10 cm, where a chord subtends a right angle at the center. This setup creates two distinct areas of interest: the minor segment and the major sector. Understanding the relationship between the circle’s radius, the subtended angle, and the areas of these segments requires a combination of circle geometry and trigonometry. This type of problem is common in fields like architecture, engineering, and design, where precise calculations are crucial.
Understanding the Geometry of the Circle
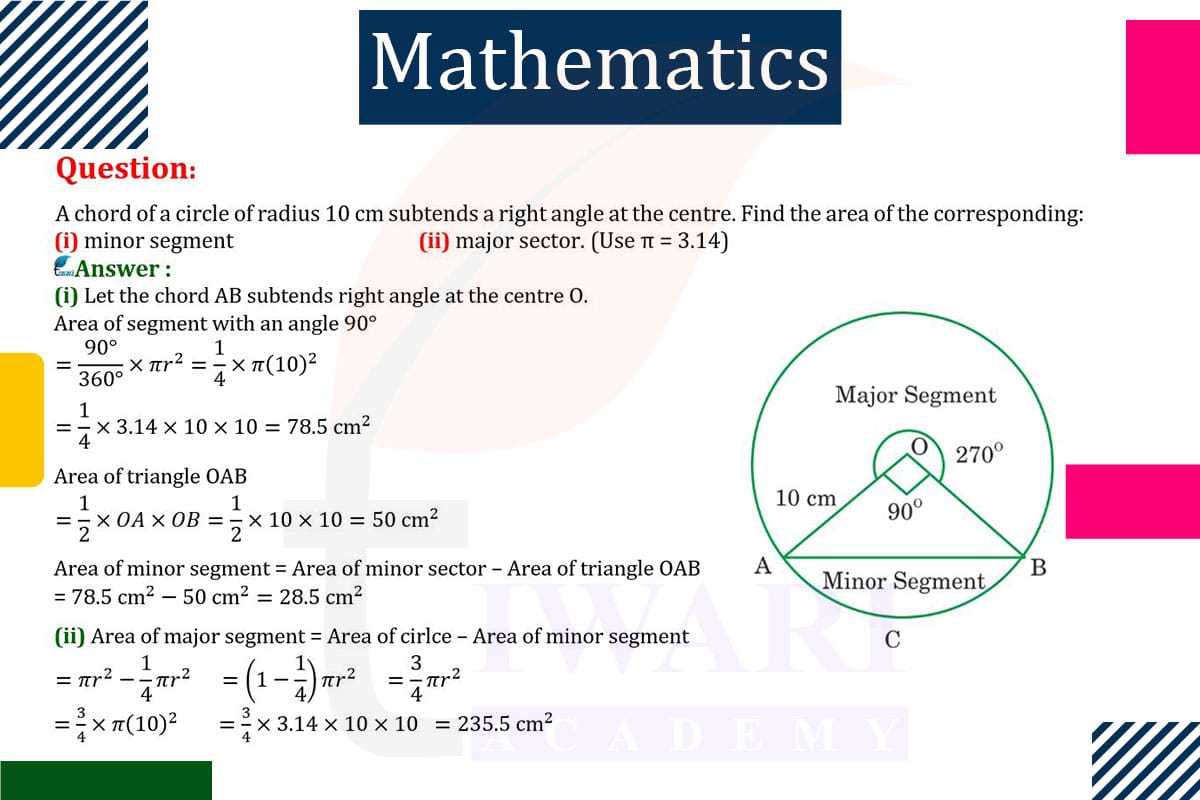
The circle in question has a radius of 10 cm. A chord that subtends a right angle at the center divides the circle into a minor segment and a major sector. The subtended right angle indicates that the sector formed is a quarter of the entire circle, and the triangle formed by the chord and the radii is a right-angled isosceles triangle. These geometric properties are key to calculating the areas of the minor segment and the major sector. The circle’s radius provides the necessary measurement to start these calculations.
Calculating the Area of the Minor Segment
To calculate the area of the minor segment, we first find the area of the sector formed by the 90° angle and then subtract the area of the right-angled isosceles triangle. The area of the sector is a quarter of the circle’s total area, given by (1/4)×π×r², where r is the radius. For a radius of 10 cm, this calculation yields 25π cm². The triangle’s area, being half the product of its base and height (both equal to the radius), is 50 cm². Thus, the minor segment’s area is 25π − 50 cm².
Determining the Area of the Major Sector
The major sector comprises the remaining part of the circle outside the minor segment. Its area can be calculated by subtracting the area of the minor segment from the total area of the circle. The total area of the circle is π×r², which equals
100π cm² for a radius of 10 cm. Subtracting the area of the minor segment from this total gives the area of the major sector. Therefore, the major sector’s area is 100π − (25π−50) cm², simplifying to 75π + 50 cm².
Practical Applications of These Calculations
Calculating the areas of segments and sectors of circles has practical applications in various fields. For instance, in engineering and architecture, these calculations are essential for designing circular parts and structures. In fields like astronomy and physics, understanding the geometry of circles is crucial for modeling celestial movements and phenomena. These calculations also have educational importance, helping students understand the practical applications of geometry in solving real-world problems.
The Interplay of Geometry and Real-World Problems
In conclusion, the problem of finding the areas of the minor segment and major sector of a circle with a given radius and chord demonstrates the interplay between geometry and practical problem-solving. It highlights the importance of understanding geometric principles and their applications in various fields. These calculations are not just theoretical exercises; they have real-world implications and are essential in many professional fields. This example serves as a testament to the relevance and utility of geometry in our daily lives and professional endeavors.
Discuss this question in detail or visit to Class 10 Maths Chapter 11 for all questions.
Questions of 10th Maths Exercise 11.1 in Detail

