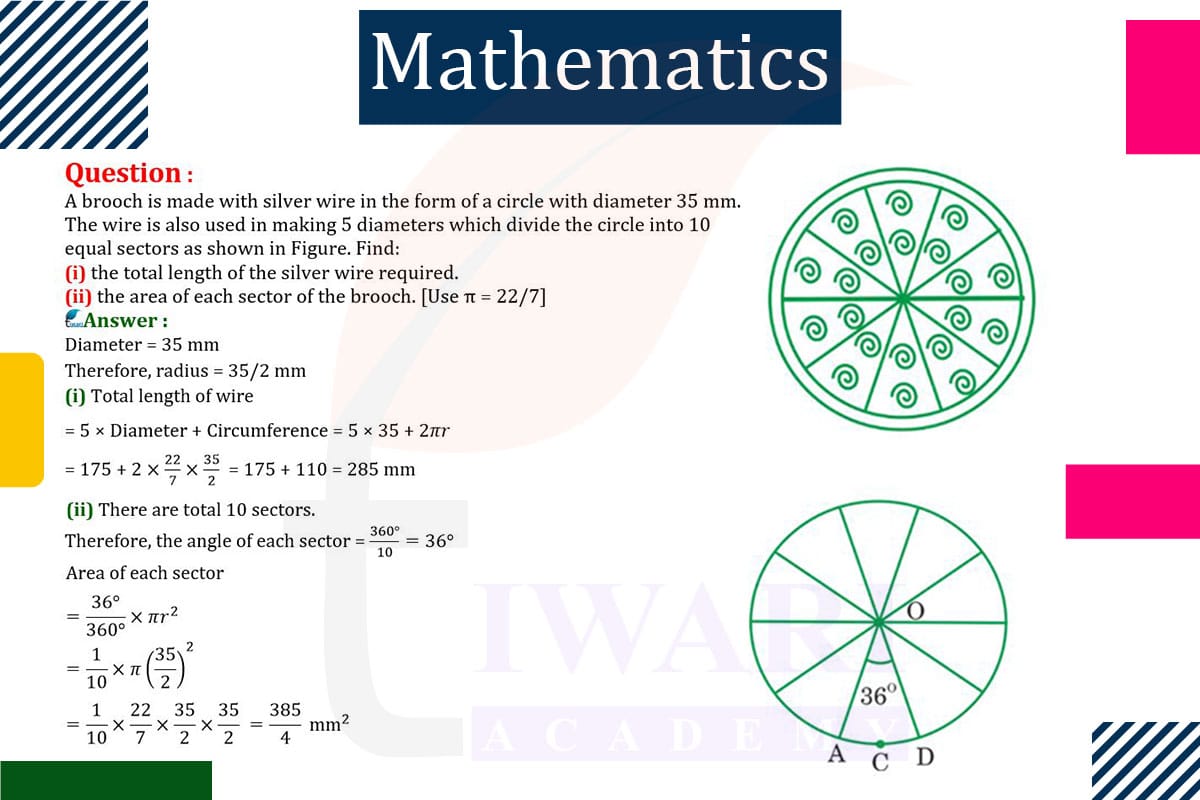
Total Length of Silver Wire:
The circumference of the circle is πd, where d = 35 mm. So, the circumference is π×35 mm. Each diameter is 35 mm, and there are 5 diameters, so the total length of the diameters is 5×35 mm. The total length of the wire is the sum of the circumference and the lengths of the diameters: π×35+5×35 mm.
Area of Each Sector:
The area of the circle is πr², where r = 17.5 mm (half of the diameter). The circle is divided into 10 equal sectors, so the area of each sector is 1/10 of the circle’s area: (1/10)×π×17.5² mm².
These calculations provide the total length of the wire needed and the area of each sector in the brooch.
Let’s discuss in detail
Introduction to Geometric Design in Jewelry Making
The creation of a brooch using silver wire, shaped into a circle with additional diameters, is a beautiful example of the application of geometry in jewelry design. In this problem, we are tasked with determining the total length of silver wire required to create a circle with a diameter of 35 mm, along with five diameters that divide the circle into ten equal sectors. Additionally, we need to calculate the area of each of these sectors. This problem not only highlights the intersection of art and mathematics but also showcases the practical applications of geometric principles in crafting intricate designs.
Calculating the Circumference of the Circle
The first step in determining the total length of silver wire needed is to calculate the circumference of the circle. The circumference of a circle is given by the formula C = πd, where d is the diameter. In our case, the diameter of the circle is 35 mm. Therefore, the circumference is π×35 mm. This calculation provides the length of the wire needed to form the outer edge of the brooch, which is the foundational element of the design.
Adding the Lengths of the Diameters
In addition to the circumference, the design includes five diameters that divide the circle into ten equal sectors. Each diameter is also 35 mm long, the same as the circle’s diameter. Therefore, the total length of the wire used for the diameters is 5×35 mm. This length must be added to the circumference to get the total length of the silver wire required for the entire brooch. This step is crucial as it incorporates the internal structure of the brooch into the total wire length calculation.
Total Length of Silver Wire Required
To find the total length of silver wire needed for the brooch, we add the circumference of the circle to the combined length of the five diameters. The total length is therefore π×35+5×35 mm. This total length encompasses both the external circle and the internal diameters, providing a complete measure of the wire needed for the entire design. This calculation is essential for the jeweler to know the amount of material required for the brooch’s creation.
Determining the Area of Each Sector
The brooch’s design divides the circle into ten equal sectors. To find the area of each sector, we first calculate the area of the entire circle using the formula A = πr, where r is the radius of the circle. With a diameter of 35 mm, the radius is half of this, 17.5 mm. The area of the circle is therefore π×17.5² mm². Since the circle is divided into ten equal sectors, the area of each sector is one-tenth of the total area, or (1/10)×π×17.5² mm².
Practical Applications and Artistic Considerations
This problem demonstrates the practical applications of geometry in artistic creations like jewelry design. Understanding the geometric properties and calculations is essential for the jeweler to accurately design and create the brooch. It ensures efficient use of materials and aids in achieving the desired aesthetic effect. Moreover, this blend of mathematics and art highlights how designers and craftsmen use mathematical principles to bring intricate and beautiful designs to life.
The Intersection of Geometry and Art
In conclusion, the design of the brooch represents a perfect blend of geometry and artistry. Calculating the total length of silver wire and the area of each sector involves applying geometric formulas to achieve a precise and aesthetically pleasing design. This problem not only illustrates the importance of geometry in practical applications but also showcases the beauty that emerges when mathematical precision is applied to artistic endeavors. It is a testament to how mathematics can be used to create beauty and functionality in everyday objects.
Discuss this question in detail or visit to Class 10 Maths Chapter 11 for all questions.
Questions of 10th Maths Exercise 11.1 in Detail

