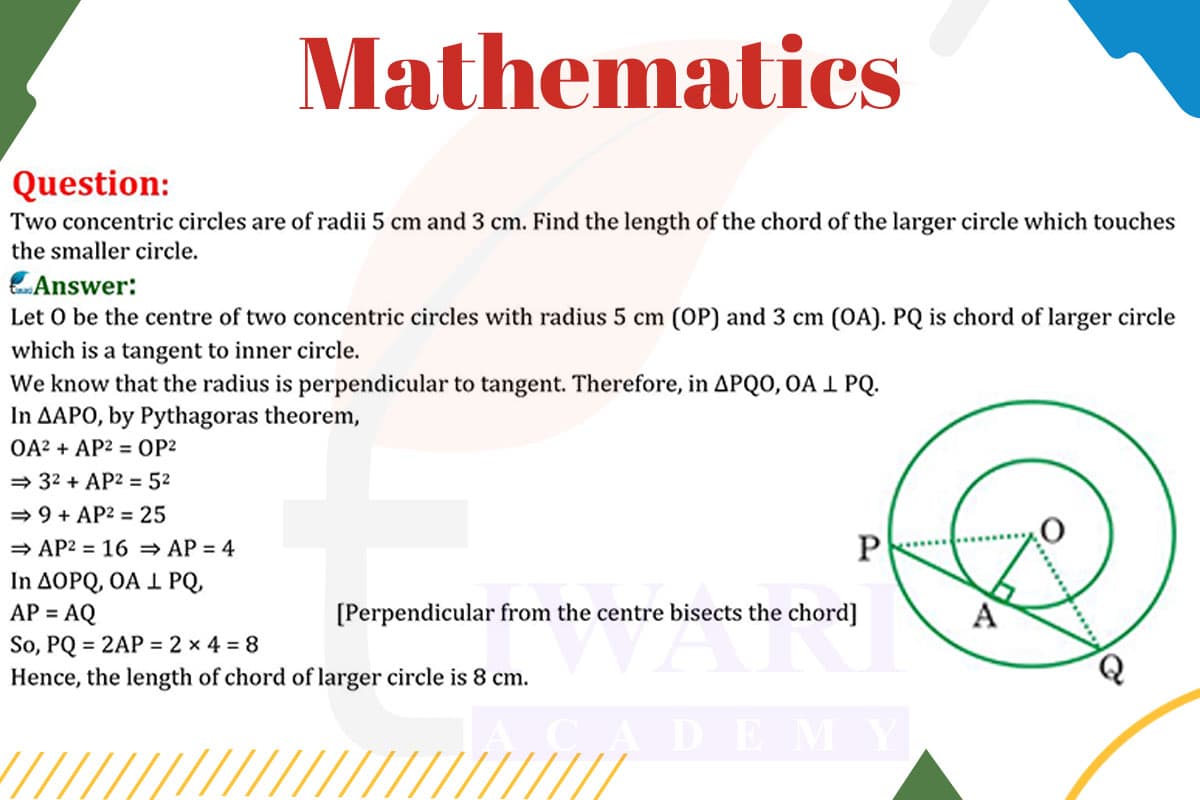
To find the length of the chord of the larger circle (radius 5 cm) that touches the smaller circle (radius 3 cm), we can use the concept of right-angled triangles.
Formation of Right-Angled Triangle: Imagine a radius of the larger circle that extends to the point of tangency on the smaller circle. This radius, the radius of the smaller circle, and half of the chord form a right-angled triangle.
Applying Pythagorean Theorem: In this triangle, the hypotenuse is the radius of the larger circle (5 cm), and one leg is the radius of the smaller circle (3 cm). The other leg is half the length of the chord.
Calculation: Let half the chord length be x. By the Pythagorean theorem, 5² = 3² + x². Simplifying, 25² = 9² + x², so x² = 16.
Chord Length: x = √16 = 4 cm. Since this is half the chord, the full chord length is 2 × 4 = 8 cm.
Therefore, the length of the chord of the larger circle that touches the smaller circle is 8 cm.
Let’s discuss in detail
Introduction to the Problem
In the study of circle geometry, problems involving concentric circles offer a unique challenge. A concentric circle is a set of two or more circles that share the same center but have different radii. A common problem in this area is to find the length of a chord of the larger circle that touches the smaller circle. This problem not only tests one’s understanding of geometric principles but also their ability to visualize and apply these principles in a slightly more complex setting than usual.
Understanding Concentric Circles
Concentric circles are circles with a common center. The beauty of concentric circles lies in their symmetry and the way they provide a framework for solving various geometric problems. In our case, we have two concentric circles with radii of 5 cm and 3 cm. The larger circle has a radius of 5 cm, while the smaller one has a radius of 3 cm. The problem at hand involves finding the length of a chord of the larger circle that just touches the smaller circle.
Visualizing the Chord and the Circles
To solve this problem, one must first visualize the chord of the larger circle that touches the smaller circle. This chord will be tangent to the smaller circle. At the point of tangency, a perpendicular can be drawn to the chord, which will be a radius of the smaller circle. This forms a right-angled triangle, with the radius of the smaller circle as one leg, the radius of the larger circle as the hypotenuse, and half of the chord length as the other leg.
Applying the Pythagorean Theorem
The Pythagorean theorem states that in a right-angled triangle, the square of the length of the hypotenuse is equal to the sum of the squares of the other two sides. In our scenario, the hypotenuse is the radius of the larger circle (5 cm), and one of the legs is the radius of the smaller circle (3 cm). The other leg is half the length of the chord, which we are trying to find.
Calculating the Chord Length
Let’s denote half the length of the chord as x. According to the Pythagorean theorem, 5² = 3² + x². Simplifying, we get 25 = 9 + x², which leads to x² = 16. Solving for x, we find x = √16 = 4 cm. Since this is half the chord, the full length of the chord is 2 × 4 = 8 cm.
Therefore, the length of the chord of the larger circle that touches the smaller circle is 8 cm. This problem is a classic example of applying the Pythagorean theorem in the context of circle geometry. It demonstrates how geometric principles can be used to solve problems that at first might seem complex but can be broken down into simpler, more manageable parts.
Discuss this question in detail or visit to Class 10 Maths Chapter 10 for all questions.
Questions of 10th Maths Exercise 10.2 in Detail

