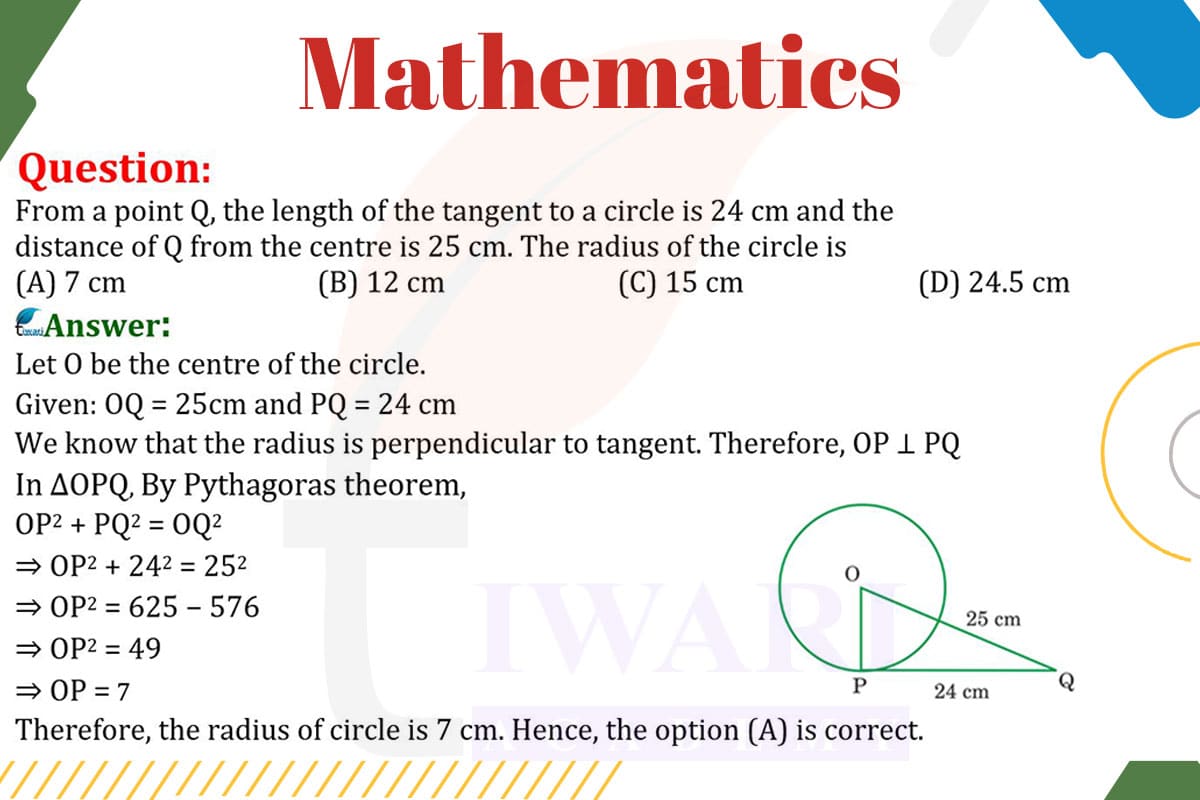
To find the radius of the circle, we can use the Pythagorean theorem. In this scenario, we have a right-angled triangle formed by the radius of the circle, the tangent from point Q to the circle, and the line segment from Q to the center of the circle.
The length of the tangent (from point Q to the point of tangency on the circle) is given as 24 cm.
The distance from Q to the center of the circle is 25 cm.
The radius of the circle (which we need to find) is the third side of the right-angled triangle.
In a right-angled triangle, the Pythagorean theorem states that the square of the hypotenuse (the side opposite the right angle, which in this case is the line segment from Q to the center) is equal to the sum of the squares of the other two sides.
Let r be the radius of the circle. Then, according to the Pythagorean theorem:
25² = 24² + r²
625 = 576 + r²
r² = 625 − 576
r² = 49
r = √49
r = 7 cm
r = 7 cm
Therefore, the radius of the circle is 7 cm.
Let’s discuss in detail
Introduction to the Problem
In geometric problems involving circles and tangents, one often encounters scenarios where the relationship between the radius of the circle, the length of the tangent from an external point, and the distance from this point to the circle’s center is explored. A classic example of such a problem is determining the radius of a circle given the length of a tangent from an external point and the distance from this point to the circle’s center. This problem not only tests one’s understanding of basic geometric principles but also their ability to apply the Pythagorean theorem in a practical context.
Understanding the Given Data
In the problem presented, we have two key pieces of information: the length of the tangent from a point Q to the circle is 24 cm, and the distance from Q to the center of the circle is 25 cm. These two data points are crucial as they form two sides of a right-angled triangle. The point where the tangent touches the circle forms a right angle with the radius at that point. This right angle is fundamental to applying the Pythagorean theorem, which is central to solving this problem.
The Role of the Pythagorean Theorem
The Pythagorean theorem is a cornerstone of geometry, stating that in a right-angled triangle, the square of the length of the hypotenuse (the side opposite the right angle) is equal to the sum of the squares of the other two sides. In our scenario, the hypotenuse is the line segment from point Q to the center of the circle, and the other two sides are the radius of the circle and the length of the tangent from Q to the circle.
Calculating the Radius
To find the radius of the circle, we set up an equation based on the Pythagorean theorem. The square of the distance from Q to the center (25 cm) equals the sum of the squares of the radius (unknown) and the length of the tangent (24 cm). Mathematically, this is represented as 25² = r² + 24². Solving this equation will give us the value of the radius.
Solving the Equation
Substituting the known values into the equation, we get 625 = r² + 576. Rearranging the equation to solve for r², we find r² = 625 − 576, which simplifies to r² = 49. The final step is to find the square root of 49, which yields r = 7. This calculation is straightforward but requires careful attention to ensure accuracy.
The radius of the circle, in this case, is found to be 7 cm. This problem is a classic example of applying the Pythagorean theorem in a geometric context. It demonstrates how a seemingly complex problem can be broken down into simpler parts using fundamental principles of mathematics. Such problems not only enhance one’s problem-solving skills but also deepen their understanding of how geometry is applied in various scenarios.
Discuss this question in detail or visit to Class 10 Maths Chapter 10 for all questions.
Questions of 10th Maths Exercise 10.2 in Detail

