NCERT Textbook Solutions for Class 10 Maths Chapter 1 Exercise 1.2 (Ex. 1.2 Class 10) Revised for New Session 2025-26, Real numbers in Hindi Medium and English Medium. Previous Years Board Questions and Solutions are also available in Video Format updated for 2025-26 board annual exams based on current NCERT books.
Exercise 1.2 Solutions
Exercise 1.2 Solutions in Hindi
Chapter 1 Solutions
Solutions of Chapter 1 Assignments
Chapter 1 MCQ Solutions
Chapter 1 Case Study MCQ
Class 10 Maths Chapter 1 Exercise 1.2 Board Questions Solutions
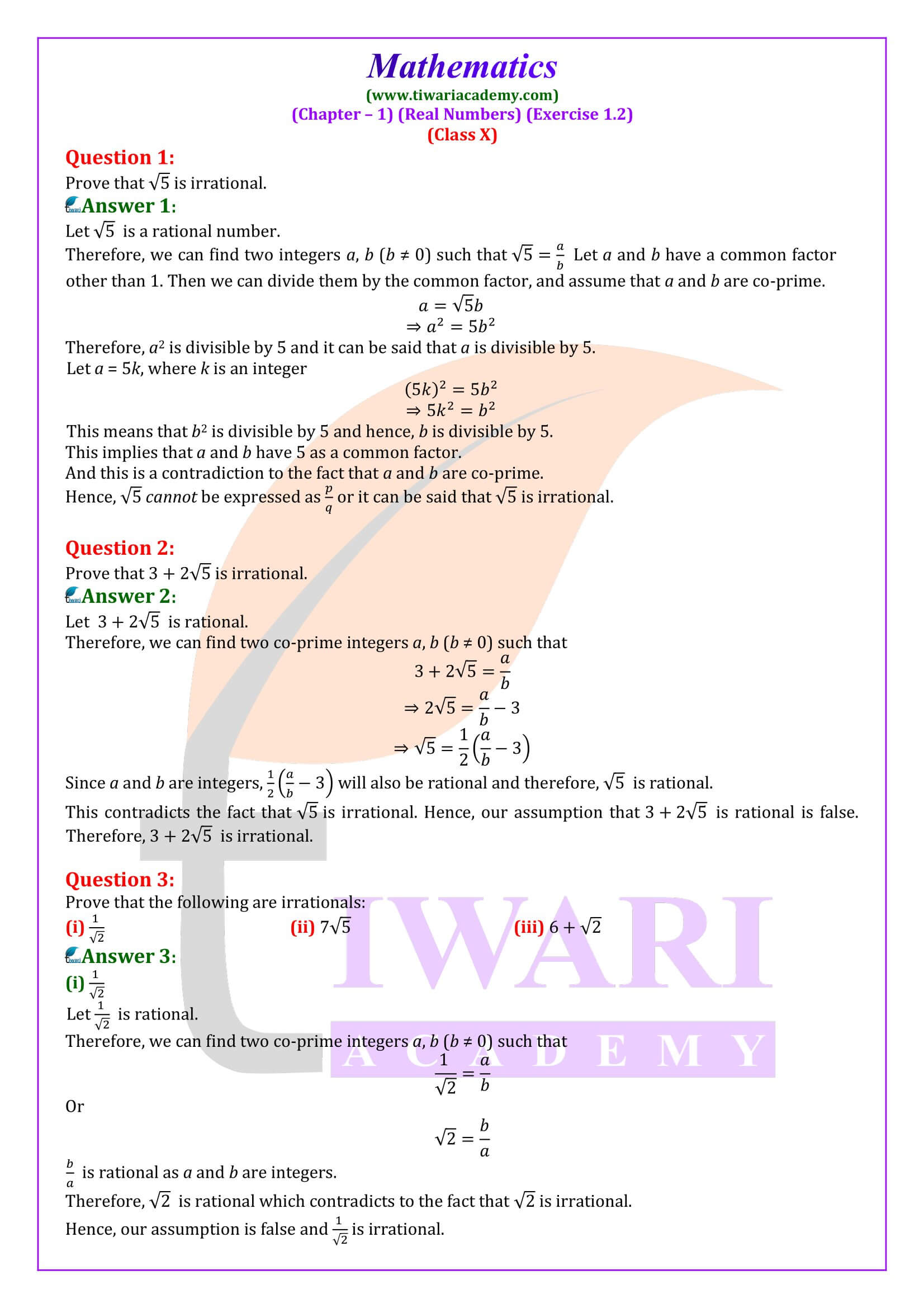
1. √4 + √5 is a rational number. Write true or false and justify your answer.
See SolutionFalse.
Justification: √4 + √5 = 2 + √5
Since 2 is rational and √5 is irrational, their sum 2 + √5 is irrational.
This is because if 2 + √5 were rational, then √5 = (2 + √5) – 2 would also be rational (as the difference of two rational numbers is rational).
But we know √5 is irrational, which creates a contradiction.
Therefore, √4 + √5 = 2 + √5 is irrational, not rational.
2. Given that √3 is irrational, show by contradiction that the sum of √3 and 2 is irrational. Show your steps.
See SolutionLet’s prove by contradiction that √3 + 2 is irrational.
Step 1: Assume that √3 + 2 is rational. If √3 + 2 is rational, it can be expressed as a fraction p/q where p and q are integers with q ≠ 0.
Step 2: If √3 + 2 = p/q, then √3 = p/q – 2 = (p – 2q)/q
Step 3: Since p and q are integers, (p – 2q)/q is also a rational number.
Step 4: This means √3 is rational, which contradicts the given fact that √3 is irrational.
Step 5: Since our assumption led to a contradiction, our original assumption must be false.
Therefore, √3 + 2 is irrational.
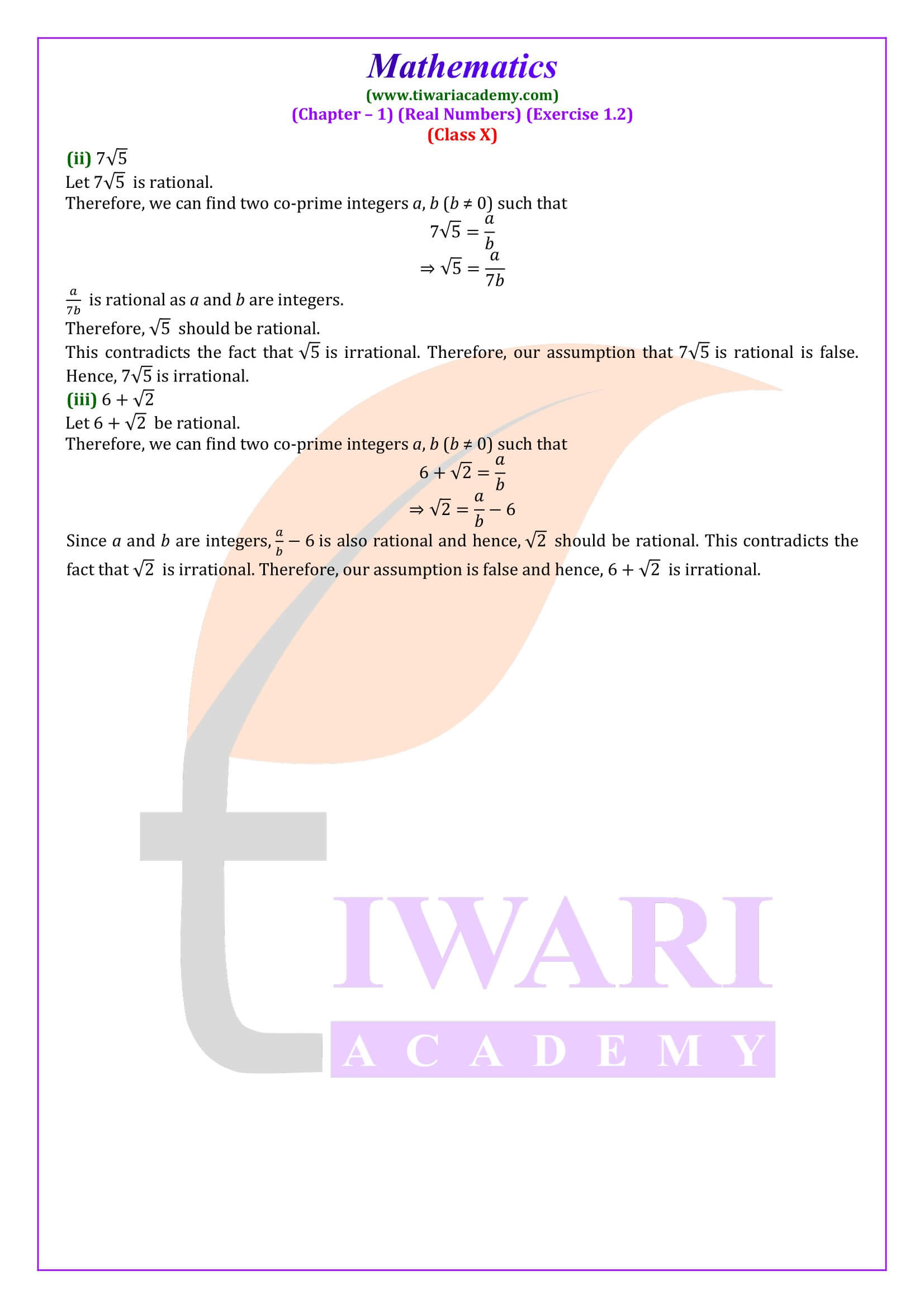
3. Prove that 1/√2 is irrational.
See SolutionI’ll prove that 1/√2 is irrational.
Step 1: Assume that 1/√2 is rational. If 1/√2 is rational, it can be expressed as a fraction p/q where p and q are integers with no common factors and q ≠ 0.
Step 2: If 1/√2 = p/q, then √2 = q/p
Step 3: Since p and q are integers, q/p is a rational number.
Step 4: This means √2 is rational, which is false (√2 is known to be irrational).
Step 5: Since our assumption led to a contradiction, our original assumption must be false.
Therefore, 1/√2 is irrational.
4. On the two real numbers a = 2 + √5 and b = 3 – √7, perform the following operations:
(i) Calculate the sum (a + b).
See Solutiona + b = (2 + √5) + (3 – √7)
= 2 + 3 + √5 – √7
= 5 + √5 – √7
(ii) Calculate the product (ab).
See Solutionab = (2 + √5)(3 – √7)
= 2(3 – √7) + √5(3 – √7)
= 6 – 2√7 + 3√5 – √5√7
= 6 – 2√7 + 3√5 – √35
= 6 + 3√5 – 2√7 – √35
(iii) Find the additive inverse of a.
See SolutionThe additive inverse of a
= 2 + √5 is -a
= -(2 + √5)
= -2 – √5
(iv) Rationalise 1/b.
See Solution1/b
= 1/(3 – √7)
= (3 + √7)/((3 – √7)(3 + √7))
= (3 + √7)/(9 – 7)
= (3 + √7)/2
(v) Verify whether the numbers a and b are rational or irrational. Provide a valid reason for your answer.
See Solutiona = 2 + √5 Since 2 is rational and √5 is irrational, their sum is irrational.
This is because if 2 + √5 were rational, then √5 = (2 + √5) – 2 would also be rational, which is a contradiction.
b = 3 – √7 Since 3 is rational and √7 is irrational, their difference is irrational.
This is because if 3 – √7 were rational, then √7 = 3 – (3 – √7) would also be rational, which is a contradiction.
Therefore, both a and b are irrational numbers.
| Class: 10 | Mathematics |
| Chapter 1: | Exercise 1.2 |
| Chapter Name: | Real Numbers |
| Content Type: | Text, Images, PDF and Videos |
| Academic Year: | Session 2025-26 |
| Medium: | English and Hindi Medium |
For NCERT Class 10 Maths Exercise 1.2, focus on proving the irrationality of numbers and understanding the application of the Fundamental Theorem of Arithmetic. The NCERT Textbook exercise 1.2 introduces proofs by contradiction, such as proving that numbers like √2 and √3 are irrational. Practice writing these proofs clearly, as they often carry significant marks in exams. Make sure you understand the logic behind each step of the proof, as questions in exams may ask you to prove the irrationality of different numbers or apply similar reasoning to new problems.
Class 10 Maths NCERT Chapter 1 Exercise 1.2 Solutions
UP Board students are also using the same NCERT Textbooks as the CBSE students. So, they also can use these solutions for their help. Here, they can download UP Board Solutions for class 10 Maths Chapter 1 Exercise 1.2 in Hindi and English Medium. Videos solutions contains the simple explanation of all the questions. NCERT Textbook Solutions Apps and website are updated according to latest CBSE or NCERT Curriculum 2025-26 for UP Board as well as CBSE Board students. Download all the digital contents in PDF or study online.
NCERT Class 10 Maths Exercise 1.2 Solutions involves understanding the relationship between the prime factorization of the denominator of a rational number and its decimal expansion, which is either terminating or repeating. Pay attention to examples provided in the chapter and practice similar problems from various resources. Going through additional solved examples and textbook explanations can help you tackle exam questions with confidence.
Important Questions of 10th Maths Exercise 1.2
HCF of 144 and 198 is (a) 9 (b) 18 (c) 6 (d) 12. [CBSE 2020] [Maths Basic]
144 = 2 x 2 x 2 x 2 x 3 x 3 = 2^4 x 3^2
198 = 2 x 3 x 3 x 11 = 2 x 3^2 x 11
HCF = 2 x 3 x 3 = 18
Hence, Option (b) is correct.
How do we find the HCF using prime factorisation?
In Prime factorisation, the HCF is the product of the smallest power of each common prime factor in the numbers.
How do we find the LCM using prime factorisation?
In Prime factorisation, the LCM is the product of the greatest power of each prime factor, involved in the numbers.
What is the relation between HCF, LCM and the two numbers?
For any two positive integers a and b, HCF (a, b) × LCM (a, b) = a × b.
NCERT Textbook 10th Maths Chapter 1 Exercise 1.2 Solutions
NCERT Exercise 1.2 Solutions for class 10 Maths Chapter 1 Real numbers in English medium as well as Hindi Medium to online use or download for offline use. If you need to download in PDF form, link is given on the page or visit Class X Maths NCERT Chapter 1 Solutions main page.
Important Questions for Practice – REAL NUMBERS
- Show that 12^n cannot end with the digit 0 or 5 for any natural number n.
- Without actual performing the long division, find if 395/10500 will have terminating or non-terminating (repeating decimal expansion.) [Answer: Non-terminating repeating]
- A rational no in its decimal expansion is 327. 7081. What can you say about the prime factors of q, when this number is expressed in the form of p/q? Give reasons. [Answer: Denominator is the multiple of 2’s and 5’s]
- What is the smallest number by which √5 – √2 is to be multiplied to make it a rational number? Also find the number so obtained? [Answer: √5 + √2, 3]
- Find one rational and one irrational no between √3 and √5. [Answer: Rational number = 1.8, Irrational number = 1.8088088808888…]
- Show that square of any odd integer is of the form 4m + 1, for some integer m.
- Show that the square of any positive integer is either of the form 4q or 4q + 1 for some integer q.
10th MATHS QUESTIONS FROM BOARD PAPERS
- Three sets of English, Hindi and Mathematics books have to be stacked in such a way that the books are stored topic wise and the height of each stack is same. The number of English books is 96, the number of Hindi books is 240 and the number of Mathematics books is 336. Assuming that the books are of same thickness, determine the number of stacks of English, Hindi and Maths books. [Answer: English = 2, Hindi = 5, Maths = 7]
- Find HCF and LCM of 56 and 112 by prime factorization method. [Answer: HCF = 56, LCM = 112]
3. Solve √45 × √20 and state what type of number is this (Rational number or irrational number). [Answer: 30, Rational number]
4. Find the HCF of 56, 96, 324 by Euclid’s algorithm. [Answer: 4]
5. Show that the cube of any positive integer is of the form 4m, 4m + 1 or 4m + 3 for some integer m.
6. Prove that √3 is an irrational number.
7. State fundamental theorem of Arithmetic and hence find the unique factorization of 120. [Answer: 2×2×2×3×5]
How many questions are there in exercise 1.2 of class 10th Maths chapter 1?
There are in all 3 questions in exercise 1.2 of class 10th mathematics chapter 1 (Real numbers) and in every question students have to prove that the given number is irrational.
How many examples are in exercise 1.2 Class 10 Maths?
3 examples are based on exercise 1.2 (chapter 1 Real numbers) of class 10th mathematics. Example 9 and question 1 are same, example 10 and questions 2, 3(iii) are of same type and example 11 and question 3(ii) are same.
What are the Important and difficult questions from exercise 1.2 of 10th Maths?
All questions of exercise 1.2 of class 10th mathematics chapter 1 (Real numbers) are important and difficulty level of questions varies from student to student. But question which most of students find little difficult is Q1.
Is exercise 1.2 of class 10th mathematics chapter 1 based on theorems?
Yes, Exercise 1.2 of class 10th mathematics chapter 1 (Real numbers) is based on Theorem 1.2 (Let p be a prime number. If p divides a^2 , then p divides a , where a is positive integer) and Theorem 1.4 (√2 is irrational).