NCERT Textbook Solutions for Class 10 Maths Chapter 1 Exercise 1.1 (Ex. 1.1) Real Numbers Modified and Updated for New Session 2025-26 in Hindi and English Medium. Board Questions and Solutions of 10th mathematics NCERT Exercise 1.1 is revised for new session 2025-26 based on new NCERT Books.
Class 10 Maths Exercise 1.1 Solutions
Class 10 Maths Exercise 1.1 in Hindi
Class 10 Maths NCERT Book Download
Class 10 Maths Chapter 1 all Exercises
Class 10 Maths Chapter 1 MCQs
Class 10 Maths NCERT Solutions
Class 10 all Subjects Solutions
Class 10 Maths Chapter 1 Exercise 1.1 From Board Papers
1. M and N are positive integers such that M = p⁵q³r² and N = p⁷q⁵r, where p, q and r are prime numbers. Find LCM(M, N) and HCF(M, N).
See SolutionTo find the LCM and HCF, we need to compare the powers of each prime factor in both numbers.
M = p⁵q³r²
N = p⁷q⁵r
For the HCF, we take the minimum power of each prime factor:
HCF(M, N) = p^min(5,7) × q^min(3,5) × r^min(2,1) = p⁵q³r
For the LCM, we take the maximum power of each prime factor:
LCM(M, N) = p^max(5,7) × q^max(3,5) × r^max(2,1) = p⁷q⁵r²
Therefore:
HCF(M, N) = p⁵q³r
LCM(M, N) = p⁷q⁵r²
2. Ajay has a box of length 3.2 m, breadth 2.4 m and height 1.6 m. What is the length of the longest ruler that can exactly measure the three dimensions of the box? Show your steps and given valid reasons.
See SolutionTo find the length of the longest ruler that can exactly measure all three dimensions, we need to find the HCF of 3.2 m, 2.4 m and 1.6 m.
First, we’ll convert these measurements to centimeters to eliminate decimals:
3.2 m = 320 cm
2.4 m = 240 cm
1.6 m = 160 cm
Now, we’ll find the HCF: HCF of 320, 240 and 160
Find the prime factorization of each number:
320 = 2⁶ × 5
240 = 2⁴ × 3 × 5
160 = 2⁵ × 5
Take the minimum power of each common prime factor:
HCF = 2^min(6,4,5) × 5^min(1,1,1) = 2⁴ × 5 = 16 × 5 = 80
Therefore, the longest ruler that can exactly measure all three dimensions of the box is 80 cm or 0.8 m.
3. 2ⁿ is not divisible by 6 where n is a positive integer. Is the above statement true or false? If true, give a valid reason and if false, give an example.
See SolutionFalse.
Example: Let n = 1, then 2¹ = 2, which is not divisible by 6. However, let n = 4, then 2⁴ = 16, which is not divisible by 6. But let n = 5, then 2⁵ = 32, which is not divisible by 6.
In fact, we can prove that 2ⁿ is never divisible by 6 for any positive integer n.
For 2ⁿ to be divisible by 6, it must be divisible by both 2 and 3.
2ⁿ is always divisible by 2 for n ≥ 1
But 2ⁿ is never divisible by 3 for any positive integer n
Since 2ⁿ is never divisible by 3, it can never be divisible by 6.
Therefore, the statement “2ⁿ is not divisible by 6 where n is a positive integer” is true.
I apologize for the error in my initial reasoning. The statement is actually true.
4. The prime factorisation of a natural number k is (3 × 5 × p) where p ≠ 2. What is the prime factorisation of 10 k²?
See SolutionThe prime factorization of k is 3 × 5 × p, where p ≠ 2.
To find the prime factorization of 10k², we’ll first find the prime factorization of 10:
10 = 2 × 5
Now, we’ll compute 10k²: 10k² = 2 × 5 × (3 × 5 × p)²
= 2 × 5 × (3 × 5 × p) × (3 × 5 × p)
= 2 × 5 × 3² × 5² × p²
= 2 × 3² × 5³ × p²
Therefore, the prime factorization of 10k² is 2 × 3² × 5³ × p².
5. A rectangular arrangement of pens has rows and columns. Rohan takes away 3 rows of pens and then Sarah takes away 2 columns of pens from the remaining pens. The remaining pens are rearranged in p rows and q columns where p is a prime number. If Rohan takes 24 pens and Sarah takes 18 pens, find all possible value(s) of p. Show your work.
See SolutionLet’s denote the original arrangement as having r rows and c columns. Total number of pens = r × c
Step 1: Rohan takes away 3 rows of pens. Number of pens Rohan takes = 3 × c = 24
Therefore, c = 8 (columns)
After Rohan takes the pens, the remaining arrangement has (r – 3) rows and 8 columns.
Step 2: Sarah takes away 2 columns from the remaining pens.
Number of pens Sarah takes = (r – 3) × 2 = 18
Therefore, r – 3 = 9, which means r = 12 (rows)
So the original arrangement had 12 rows and 8 columns.
Step 3: After both Rohan and Sarah take pens, the remaining arrangement has (r – 3) rows and (c – 2) columns. That’s 9 rows and 6 columns.
Step 4: These pens are rearranged into p rows and q columns, where p is prime. Number of remaining pens = 9 × 6 = 54
The factors of 54 are: 1, 2, 3, 6, 9, 18, 27, 54
For p rows and q columns, p × q = 54 and p must be prime.
The possible values of p (prime factors of 54) are: 2, 3
Therefore, p could be either 2 or 3.
The possible arrangements are:
p = 2, q = 27
p = 3, q = 18
The possible values of p are 2 and 3.
| Class: 10 | Mathematics |
| Chapter 1: | Exercise 1.1 |
| Topic: | Real Numbers |
| Content: | Exercise Solutions and Extra Questions |
| Session: | Academic Year 2025-26 |
| Medium: | English and Hindi Medium |
For Exercise 1.1 in Chapter 1 of the NCERT Class 10 Maths Real Numbers solutions, preparation should focus on mastering the prime factorisation method and calculating the HCF and LCM of numbers. Start by thoroughly practicing the problems where numbers are expressed as products of prime factors. Familiarise yourself with the steps involved in determining the HCF and LCM using the prime factorisation method, as this is useful for solving related questions in exams. Ensure to verify your solutions by confirming that the product of the HCF and LCM equals the product of the given numbers. Practicing these types of problems repeatedly will build speed and accuracy, which are essential for exams.
Pay special attention to word problems, such as the one where two individuals start at the same point and you need to calculate when they will meet again using LCM. Such problems often appear in exams, testing both your conceptual understanding and problem-solving skills. Make a list of important formulas, like the relation between HCF and LCM, and revise them regularly. Solving sample papers and previous year questions will help you get used to the type of questions asked and the level of difficulty.
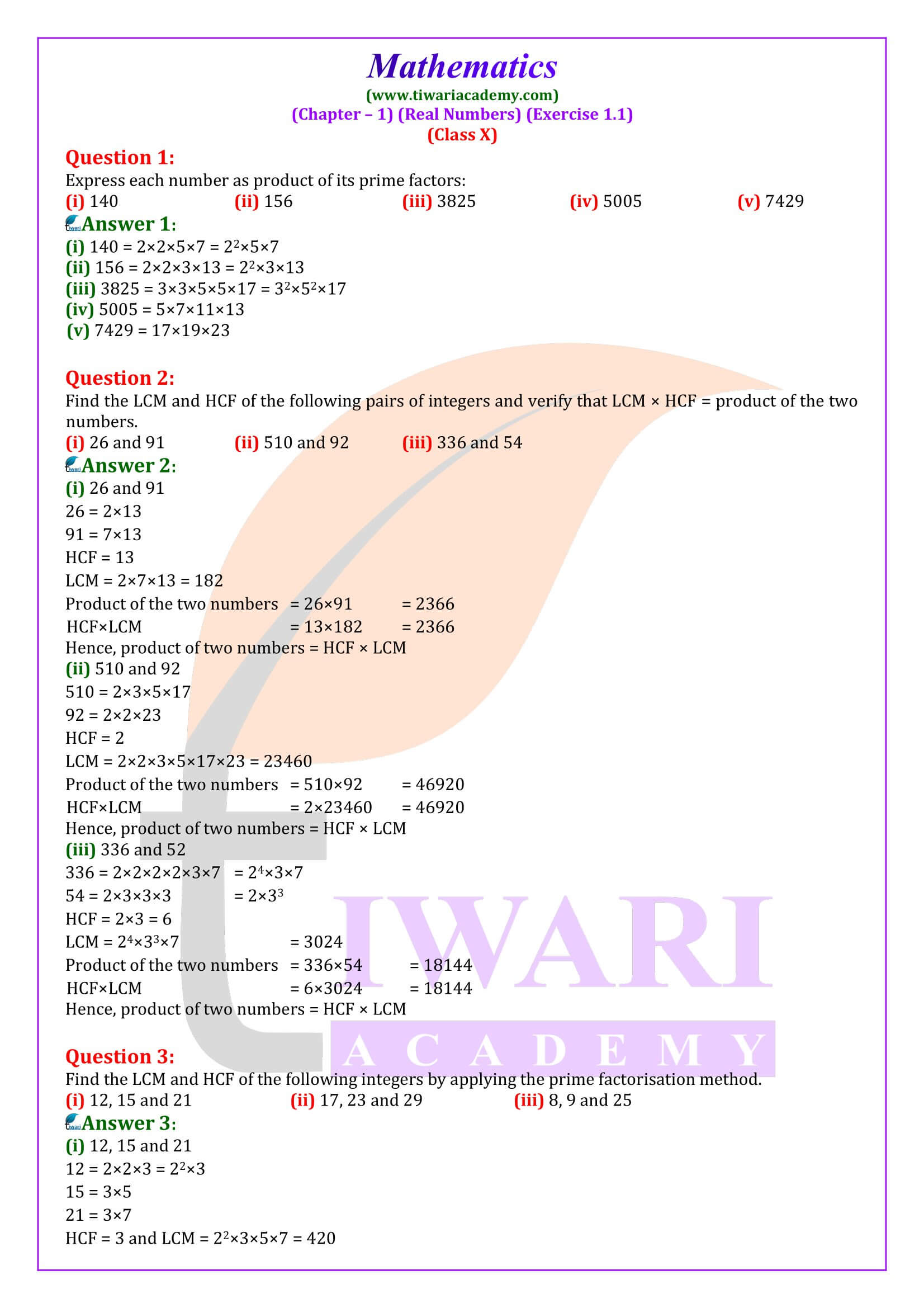
Class 10 Maths NCERT Chapter 1 Exercise 1.1 Question 1
Express each number as product of its prime factors:
- (i) 140
- (ii) 156
- (iii) 3825
- (iv) 5005
- (v) 7429
Solution:
(i) 140 = 2 × 2 × 5 × 7 = 2² × 5 × 7
Discuss this question in your opinion.
(ii) 156 = 2 × 2 × 3 × 13 = 2² × 3 × 13
If you have any other suggestion, please suggest us.
(iii) 3825 = 3 × 3 × 5 × 5 × 17 = 3² × 5² × 17
More options related to above question.
(iv) 5005 = 5 × 7 × 11 × 13
if you have any other idea, please comment here.
(v) 7429 = 17 × 19 × 23
What do you think about 10th Maths Exercise 1.1 Part v solutions?
Class 10 NCERT Maths Chapter 1 Exercise 1.1 Question 2
Find the LCM and HCF of the following pairs of integers and verify that LCM × HCF = Product of the two numbers.
- (i) 26 and 91
- (ii) 510 and 92
- (iii) 336 and 54
Solution:
(i) 26 and 91
26 = 2 × 13
91 = 7 × 13
HCF = 13
LCM = 2 × 7 × 13 = 182
Product of the two numbers = 26×91 = 2366
HCF × LCM = 13 × 182 = 2366
Hence, product of two numbers = HCF × LCM
Do you have more ideas about this solutions?
(ii) 510 and 92
510 = 2 × 3 × 5 × 17
92 = 2 × 2 × 23
HCF = 2
LCM = 2 × 2 × 3 × 5 × 17 × 23 = 23460
Product of the two numbers = 510 × 92 = 46920
HCF × LCM = 2 × 23460 = 46920
Hence, product of two numbers = HCF × LCM
You can discuss this question with us.
(iii) 336 and 54
336 = 2 × 2 × 2 × 2 × 3 × 7 = 2⁴ × 3 × 7
54 = 2 × 3 × 3 × 3 = 2 × 3³
HCF = 2 × 3 = 6
LCM = 2⁴ × 3³ × 7 = 3024
Product of the two numbers = 336 × 54 = 18144
HCF × LCM = 6 × 3024 = 18144
Hence, product of two numbers = HCF × LCM
Comment about the solution here.
NCERT Class 10 Maths Chapter 1 Exercise 1.1 Question 3
Find the LCM and HCF of the following integers by applying the prime factorisation method.
- (i) 12, 15 and 21
- (ii) 17, 23 and 29
- (iii) 8, 9 and 25
Solution:
(i) 12, 15 and 21
12 = 2 × 2 × 3 = 2² × 3
15 = 3 × 5
21 = 3 × 7
HCF = 3
LCM = 2² × 3 × 5 × 7 = 420
For more suggestion, see the solution here.
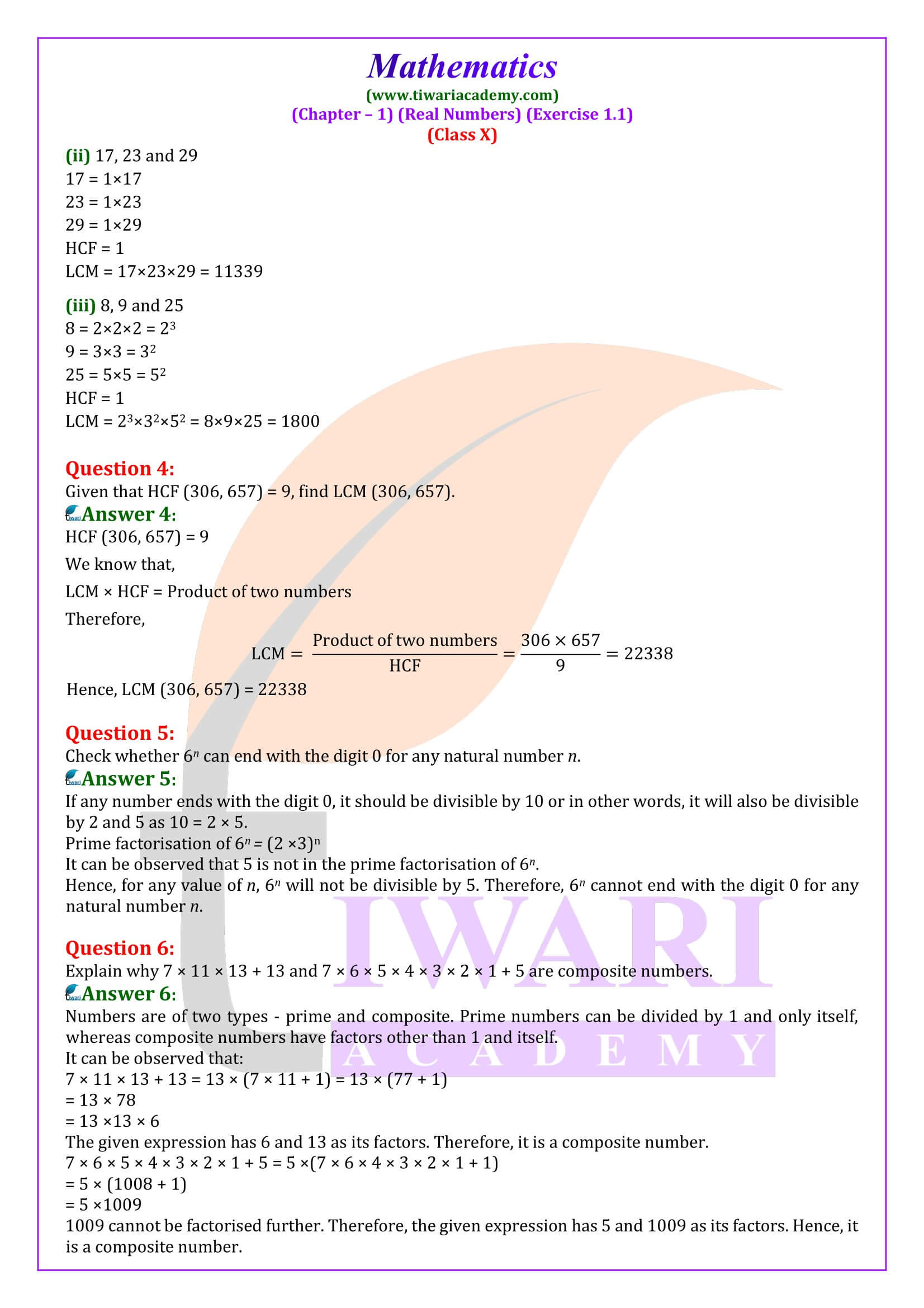
(ii) 17, 23 and 29
17 = 1 × 17
23 = 1 × 23
29 = 1 × 29
HCF = 1
LCM = 17 × 23 × 29 = 11339
Prove more ways to solve this question.
(iii) 8, 9 and 25
8 = 2 × 2 × 2 = 2³
9 = 3 × 3 = 3²
25 = 5 × 5 = 5²
HCF = 1
LCM = 2³ × 3² × 5² = 8 × 9 × 25 = 1800
How can we solve this question alternatively?
Class 10 Maths NCERT Chapter 1 Exercise 1.1 Question 4
Given that HCF (306, 657) = 9, find LCM (306, 657).
Solution:
HCF (306, 657) = 9
We know that,
LCM × HCF = Product of two numbers
Therefore,
LCM= (Product of two numbers)/HCF = (306 × 657)/9 = 22338
Hence, LCM (306, 657) = 22338
The alternative way to solve this question.
Class 10 Maths Chapter 1 Exercise 1.1 NCERT Question 5
Check whether 6ⁿ can end with the digit 0 for any natural number n.
Solution:
If any number ends with the digit 0, it should be divisible by 10 or in other words, it will also be divisible by 2 and 5 as 10 = 2 × 5.
Prime factorisation of 6ⁿ = (2 × 3)ⁿ
According to Fundamental Theorem of Arithmetic, the factorisation is uniqe. It can be observed that 5 is not in the prime factorisation of 6ⁿ.
Hence, for any value of n, 6n will not be divisible by 5. Therefore, 6n cannot end with the digit 0 for any natural number n.
Put your opinion about question 5 of 10th Maths exercise 1.1
Class 10 NCERT Math Chapter 1 Exercise 1.1 Question 6
Explain why 7 × 11 × 13 + 13 and 7 × 6 × 5 × 4 × 3 × 2 × 1 + 5 are composite numbers.
Solution:
Numbers are of two types – prime and composite. Prime numbers can be divided by 1 and only itself, whereas composite numbers have factors other than 1 and itself.
It can be observed that:
7 × 11 × 13 + 13 = 13 × (7 × 11 + 1) = 13 × (77 + 1)
= 13 × 78
= 13 × 13 × 6
The given expression has 6 and 13 as its factors. Therefore, it is a composite number.
Now: 7 × 6 × 5 × 4 × 3 × 2 × 1 + 5 = 5 ×(7 × 6 × 4 × 3 × 2 × 1 + 1)
= 5 × (1008 + 1)
= 5 × 1009
1009 cannot be factorised further. Therefore, the given expression has 5 and 1009 as its factors. Hence, it is a composite number.
For any further suggestion, please visit here.
NCERT Class 10 Mathematics Chapter 1 Exercise 1.1 Question 7
There is a circular path around a sports field. Sonia takes 18 minutes to drive one round of the field, while Ravi takes 12 minutes for the same. Suppose they both start at the same point and at the same time, and go in the same direction. After how many minutes will they meet again at the starting point?
Solution:
It can be observed that Ravi takes lesser time than Sonia for completing 1 round of the circular path. As they are going in the same direction, they will meet again at the same time when Ravi will have completed 1 round of that circular path with respect to Sonia. And the total time taken for completing this 1 round of circular path will be the LCM of time taken by Sonia and Ravi for completing 1 round of circular path respectively i.e., LCM of 18 minutes and 12 minutes.
18 = 2 × 3 × 3
And, 12 = 2 × 2 × 3
LCM of 12 and 18 = 2 × 2 × 3 × 3 = 36
Therefore, Ravi and Sonia will meet together at the starting point after 36 minutes.
What do you think about the solution of question 7 given in Ex. 1.1 of 10th Maths.
Important questions for 10th Maths Exercise 1.1
What is fundamental theorem of Arithmetic?
According to fundamental theorem of arithmetic:
Every composite number can be expressed ( factorised) as a product of primes, and this factorisation is unique, apart from the order in which the prime factors occur.
225 can be expressed as (a) 5 x 3^2 (b) 5^2 x 3 (c) 5^2 x 3^2 (d) 5^3 x 3. [CBSE 2020] [Maths Basic]
225 = 3 x 3 x 5 x 5 = 3^2 x 5^2.
Hence, Option (c) is correct.
The total number of factors of a prime number is (a) 1 (b) 0 (c) 2 (d) 3. [CBSE 2020] [Maths Standard]
The total number of factors of a prime number is 2. One is 1 and other is itself number.
Hence, Option (c) is correct.
The HCF and the LCM of 12, 21, 15 respectively are (a) 3, 140 (b) 12, 120 ((c) 3, 420 (d) 420, 3 [CBSE 2020] [Maths Standard]
12 = 2 x 2 x 3
21 = 3 x 7
15 = 3 x 5
HCF = 3
LCM = 2 x 2 x 3 x 7 x 5 = 420
Hence, Option (c) is correct.
NCERT 10th Maths Chapter 1 Exercise 1.1 Solutions
NCERT Updated Solutions for class 10 Mathematics Chapter 1 Exercise 1.1 Real Numbers in English medium as well as Hindi Medium is given below to free download in PDF format for 2025-26. IF YOU WANT TO DOWNLOAD, THE LINK IS ALSO GIVEN or visit NCERT Solutions for Class 10 Math NCERT Chapter 1 to download other exercises.
Important Questions on REAL NUMBERS
- In a seminar, the number of participants in English, Maths and Science are 175, 140 and 105, respectively. Find the minimum number of rooms required if in each room the same number of participants are to be seated and all of them being in the same subject. [Answer: 12]
- Find the value of m if HCF of 65 and 117 is expressible in the form 65m – 117. [Answer: 2]
- What can you say about the product of a non-zero rational and irrational number? [Answer: Irrational]
- In a school there are two sections of class X – section A and section B. There are 48 students in sections A and 54 students in section B. Determine the least number of books required for the library of the school so that the books can be distributed equally among students of section A or section B? [Answer: 216]
- After how many places the decimal expansion of 13497/1250 will terminate? [Answer: 4]
- Find the least number which is divisible by all numbers from 1 to 10 (both inclusive). [Answer: 2520]
Practice Questions on Real Numbers
- A circular field has a circumference of 360 km. Three cyclists start together and can cycle 48 km, 60 km and 72 km a day, round the field. After how many days will they meet again at the starting point? [Answer: 30 days]
- The numbers 525 and 3000 are divisible by 3, 5, 15, 25 and 75 what is the HCF of 525 and 3000? [Answer: 75]
- Can two numbers have 18 as their HCF and 380 as their LCM? Give reasons. [Answer: No]
- If a = 4q + r then what are the condition for a and q? What are the values that r can take? [Answer: a and q are positive integer 0 ≤ r ≤ 4]
- What is the digit at unit’s place of 9^n? [Answer: Even power = 1, odd power = 9]
- If n is an odd integer then show that n² – 1 is divisible by 8.
- Use Euclid’s division algorithm to find the HCF of 16 and 28. [Answer: 4]
Ask your doubts related to NIOS or CBSE Board and share your knowledge with your friends and other users through Discussion Forum.
How many questions are there in Class 10 Maths Exercise 1.1?
There are in all 7 questions in exercise 1.1 of class 10th mathematics chapter 1 (Real numbers) and every question is different from each other.
Which example is important for exercise 1.1 of 10th mathematics?
4 examples are based on exercise 1.1 (chapter 1 Real numbers) of class 10th mathematics. Example 5 and question 5 are based on same concept, example 7 and question 4 are based on same formula and examples 6, 8 and question 3 are same. All the examples are important for the exams as well as school tests.
What are the Important and difficult questions from exercise 1.1 in 10th Maths?
Question number 2, 4, 5 and 7 of exercise 1.1 of class 10th mathematics chapter 1 (Real numbers) are important and difficulty level of questions varies from student to student.
But questions which most of students find little difficult in this exercise are Q5, 6 and 7.
Which topics students should recall before starting exercise 1.1 of 10th Maths?
Before starting exercise 1.1 (chapter 1 Real numbers) of class 10th mathematics, Students should recall meaning of:
Factors.
HCF (Highest Common Factor) and LCM (Lowest/Least Common Multiple).
Prime numbers, Composite numbers, Natural numbers.